The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
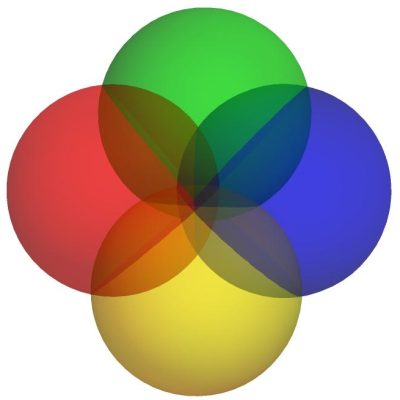
Binding energy as intersection of spacetime density.
Fine structure constant α
leer
To define the fine structure constant, the term “force” must be clarified. Without a force, all objects remain constant in their states of motion. Therefore, force means the change of a DRD. The gravitational constant G is the proportionality constant for the calculation of the force. Thus, G represents the resistance of spacetime to intrinsic change and is defined in value as the higher dimensional limit of our spacetime.
For the fine structure constant, the force must be considered at an electromagnetic interaction. This lies, because of the photon as exchange particle in the 2D. Therefore, for a comparison of the forces between the gravity and the electromagnetic interaction, the following two conditions result:
- DThe electromagnetic force must be much larger than the gravitation. A spacetime with only two spacedimensions is easier to change than a spacetime with three spacedimensions. The difference for the charge and rest mass of two electrons is about 4\space *\space 10^{42}. The hierarchy problem is “only” the dimensional transition.
- In interaction, the photon between two 2D objects generates a force which is measured in 3D. This must be smaller, because the photon again represents a gravitation, which weakens the DRD. This difference is the fine structure constant.
The fine structure constant is the difference of the force between an E-field and a G-field at the limit of the spacetime structure. Therefore, for a comparison of the forces, we choose the respective maximum conditions.
Gravitational force is given by F\space =\space \frac{G\space *\space m_1\space *\space m_2}{r^2}.
Maximum mass of a single object is the Planck mass and thus F\space =\space \frac{G\space *\space m_P^2}{r^2}.
The force in the electric field is given by F\space =\space \frac{1}{4\space *\space \pi\space *\space \epsilon_0}\space *\space \frac{e\space *\space e}{r^2} .
Maximum charge of a single object is the elementary charge and thus F\space =\space \frac{1}{4\space *\space \pi\space*\space \epsilon_0}\space *\space \frac{e^2}{r^2} .
The two forces are put into the ratio
\cfrac{\cfrac{1}{4\space *\space \pi\space*\space \epsilon_0}\space *\space \cfrac{e^2}{r^2}}{\cfrac{G\space *\space m_P^2}{r^2}}\space \iff\space \cfrac{e^2}{4\space *\space \pi\space*\space \epsilon_0\space *\space G\space *\space m_P^2}
m_P^2 (reduced) is replaced by m_P\space =\space \frac{l_P\space *\space c^2}{G}, with l_P for the Planck length.
l_P (reduced) is then replaced by l_P\space =\space \sqrt{\frac{\hbar\space *\space G}{c^3}}.
\cfrac{e^2}{4\space *\space \pi\space*\space \epsilon_0\space *\space G\space *\space (\cfrac{l_P\space *\space c^2}{G})^2}\space \iff\space \cfrac{e^2}{4\space *\space \pi\space*\space \epsilon_0\space *\space G\space *\space \cfrac{l_P^2\space *\space c^4}{G^2})}\space \iff\space \cfrac{e^2\space *\space G}{4\space *\space \pi\space*\space \epsilon_0\space *\space l_P^2\space *\space c^4}\space \iff\space \cfrac{e^2\space *\space G}{4\space *\space \pi\space*\space \epsilon_0\space *\space (\sqrt{\cfrac{\hbar\space *\space G}{c^3}})^2\space *\space c^4}\space \iff\space \cfrac{e^2\space *\space G}{4\space *\space \pi\space*\space \epsilon_0\space *\space \cfrac{\hbar\space *\space G}{c^3}\space *\space c^4}\space \iff\space \cfrac{e^2}{4\space *\space \pi\space*\space \epsilon_0\space *\space \hbar\space *\space c}
The result is α, the fine structure constant.
It follows that the force of the G-field is α larger than the force of the E-field under the given conditions. One could also have replaced m_P directly by \sqrt{\frac{\hbar\space *\space c}{G}}. In the DP, everything is normalized by the Planck length.
For a better understanding of what α represents, the result from the force comparison is transformed. The transformation is to be based on the space-time structure, as in the case of G.
\cfrac{e^2}{4\space *\space \pi\space*\space \epsilon_0\space *\space \hbar\space *\space c}\space \iff\space \cfrac{e^2}{2\space *\space \epsilon_0\space *\space h\space *\space c} transform into the unreduced variant of h
\cfrac{e^2}{2\space *\space \epsilon_0\space *\space E\space *\space t_P\space *\space \cfrac{l_P}{t_P}}\space \iff\space \cfrac{e^2}{2\space *\space \epsilon_0\space *\space E\space *\space l_P} transform into h\space =\space E\space *\space t_P und c\space =\space \cfrac{l_P}{t_P}
Split the found description into two parts
\cfrac{e\space *\space e}{2\space *\space l_P\space *\space E}\space *\space \cfrac{1}{\epsilon_0}
other representation with magnetic field strength
\cfrac{e\space *\space e}{2\space *\space l_P}\space *\space \cfrac{\mu_0}{m_P}
The first term describes how two charges act on the maximum DRD (from Planck mass), which is in two Planck lengths (change – force). The two Planck lengths are again the result from the previous section. The second term describes the behavior of the electric field constant as a proportionality contant. Resistance from a 3D point of view.
The fine structure constant α, like all other fundamental quantities, is thus bound to the spacetime limits. Since α is an attenuation of any DRD across the dimensional boundary, no units of measure are available. The electromagnetic interaction is again a gravity in 2D and thus attenuates a DRD from 3D. All objects in an electric field must be reduced by α. For each space dimension involved, α must be used once.
At very high energy, α changes its value and becomes larger. The higher the energy, the more 2D approaches 3D. From the energy of a Planck length, 2D becomes a 3D spacetime. Thus, at the maximum energy, the value of α must go to 1. Since α is composed only of natural constants, it is an interesting question which one should change? Since from the DP the values m_P, l_P or c cannot change, only two possibilities remain:
- The elementary charge: As the DRD increases, more 2D gravity fits into a small area. This would correspond to an increase in charge.
- \epsilon_0 and \mu_0 in opposite directions: the combination of both values must always result in c. However, since a higher DRD can also produce a stronger resistance in 2D, \epsilon_0 could increase. Since this can then penetrate another 2D spacetime more easily, \mu_0 could fall.
It is possible that all 3 values change. Therefore the changes are only very small per single value.