The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
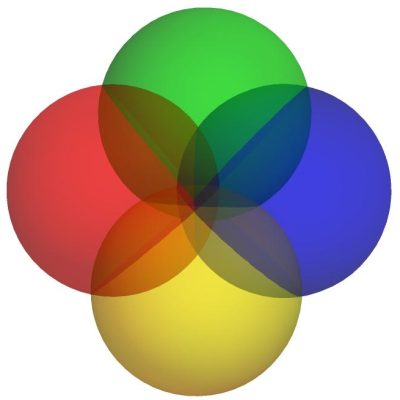
Binding energy as intersection of spacetime density.
7 Cosmology (construction site)
leer
In cosmology, as with the SM, the BHs are the central objects in our spacetime. The idea of spacetimes with different numbers of spatial dimensions must be expanded again. There are also infinitely many 3D spacetimes, which can influence each other. Dark energy (DE) and dark matter (DM) can consist of different components. In the DP, the actual origin of the universe from the Big Bang is not clarified. We assume a very small but given spacetime.
Beautiful, but not mandatory
From a purely philosophical point of view, the following train of thought is very “nice and round”, but unfortunately without a really compelling logic. Since in DP a lot depends on the spacetime structure and the BHs, one can come up with the idea that our universe is the result of an BH out of 4D. Then a 3D BH is an elementary particle in 4D and an BH in 4D is a universe in 3D. The whole thing recursively down to 2D. There are certain observations which might suggest that our universe is an BH. A 4D spacetime is much harder to change than a 3D spacetime. The total mass of the universe (with DM) would then have to correspond to the Planck mass in 4D. As nice as the idea is, we put it aside and take our spacetime as given.
What emerges from this consideration as compelling is that the Big Bang must have happened out of a higher- or lower-dimensional transition. The GR fails in the singularity of the big bang. Thus, it must not have happened out of our spacetime. The GR describes everything completely in our spacetime.
Other 3D spacetime
As a first conclusion, the Copernican principle is to be further extended to spacetime. In the previous chapters, this principle was used to refer to lower-dimensional spacetime. A higher-dimensional limit of spacetime is the singularity in an BH. Since our spacetime itself has BHs, this means that there must necessarily be at least one other 4D spacetime. Then there can be any number of other 3D spacetimes. These other 3D spacetimes must have the same physical laws as our spacetime. In the DP, the physical laws depend only on the spacetime structure. This is the same for all 3D spacetimes. This means that there are also BHs there and, in particular, their own dynamics in spacetime. Therefore, these spacetimes can influence each other. Thus, there can be structures in our spacetime that cannot be explained by the content or dynamics of our spacetime alone. This will be illustrated by part of the DM.
Very small and very early BHs
In the early universe, the DRD of the vacuum was very high. In fact, interaction can occur where an BH was created directly from a Planck mass. These BHs have an SSR of 2 Planck lengths. This makes the interaction cross-section extremely small. All other objects in the universe are much larger than these BHs. Even though many of these BHs have formed, it is very unlikely that an BH of this type will continue to grow. Only a few have managed to do so. BHs have already existed since the beginning of the universe. This process solves several problems at once:
- Some of the BHs may have merged or grown by absorbing DRD. This means that very large BHs can be observed very early in the universe.
- The BHs that could not grow (almost all of them) remain as Planck BHs. These form the second part of the DM. The BHs cannot be destroyed by Hawking radiation, because not enough particles with “small” wavelengths can be produced in the vacuum due to the strongly falling DRD. These Planck BHs are BHL corpses”.
- An BH is a connection to a higher-dimensional spacetime and also simultaneously to other 3D spacetimes. Once a connection is made, our spacetime must align with the other spacetimes. The first BH created the inflation phase. The inflation phase is the alignment with the already existing spacetimes. Since this works across dimensional boundaries, it happens almost instantaneously. As usual in DP, no other field is needed for inflation.
Let’s move on to the two most important points for cosmology. What are DM and DE?
Dunkle Materie (DM)
Dunkle Materie setzt sich aus zwei Komponenten zusammen.
Die erste Komponente sind Planck-SLs aus dem Urknall. Diese reagieren nur per Gravitation. Der WW-Querschnitt ist so klein, dass diese mit fast nichts eine WW erzeugen. Selbst wenn sich mal zwei SL vereinigen sollten, wird man keine Strahlung erkennen können und die Wirkung als DM hat sich fast nicht verändert. Alle anderen Objekte des Standardmodells sind in Ihren Compton-Wellenlängen zu groß, um von dem SL „gefressen“ werden zu können. Diese DRD ist als einzelnes „Quantum“ und um viele Größenordnungen größer als das Planck-SL selbst. Damit haben wir eine geometrische Ausprägung, die nur per Gravitation interagiert und so gut wie keine WW mit anderen Objekten hat. Dagegen sind selbst Neutrinos reaktionsfreudig.
Die zweite Komponente ist die gravitative WW mit anderen 3D-Raumzeiten. Dies funktioniert wie bei 2D wiederum nur bei einem SL. Diese Wirkung gibt es mit allen Formen eines SL in unserer Raumzeit oder mit einem SL in der anderen Raumzeit. Daher ergibt sich ein verstärkender Effekt, wenn sich ein SL gebildet hat. Zusätzlich können Zusammenhänge erzeugt werden, die es auf Grund der Größe in unserem Universum allein nicht geben kann (z.B. der Große Bogen). Es können sich Vorzugsrichtungen bilden, die es sonst nicht geben kann. Zum Beispiel die Ringstruktur der Zwerggalaxien um eine große Galaxie.
Dark matter (DM)
There are three alternatives for the DE. Due to the state of work of this version, no prioritisation has been made as to which of the alternatives is the better one. It is also possible that there are only different descriptions for the same issue or that there are mixtures.
Vacuum energy:
In the DP, vacuum energy is defined by spacetime itself. Where there is a point in space, there is also energy. Thus, particles can also form for this energy in the lower dimension. For a particle formation, however, one needs a given geometry. This means that the entire volume of spacetime does not take part in this process. Only the part that is occupied with a specific DRD. If we look at the average occupancy of the universe with DRD, we come to approx. 1 -2 atoms per , 410 photons per and 340 neutrinos per . Only these objects generate a fluctuation. Then this fluctuation comes on average only with the energy of the objects. The orders of magnitude difference in energy from vacuum to DE is nonsense. The values are not that far apart.
Disadvantage of the solution: the DE then spreads out with the expansion and would also have to dilute. This is not observed. Advantage: The Hubble constant from the supernova would then have to be larger than the measurement from the background radiation. Since here measurements are always taken from mass accumulation to mass accumulation and a mass accumulation must always have a high DE.
Gravity without mass:
In the case of photons, a gravitational fluctuation is generated in 2D. However, there is no DRD in these spacetimes. According to the field equation, this actually results in a negative mass, since this is missing. Then the photon in 2D (not the complete spacetime) would have to have a spacetime expansion away from the DRD and expand on its own. Then the universe would not explicitly expand strongly, but only the photon. There is then no rapidly expanding universe. This is only a mirage from the expansion of the single photon. Even if the universe has almost come to a standstill, the photons show a different picture.
Disadvantage: If the universe started with a big bang, it must also continue to expand. Where does the energy come from then? Only from inflation?
Cosmological constant:
The field equation describes the effects of gravity very precisely even without the constant. The constant is only needed if one wants to apply the field equation to the universe as a whole. Then it can create a global balance and represent a certain scenario. At present, an expanding universe is needed.
If one sets the energy-momentum tensor to zero for the field equation, the Einstein tensor disappears. Only this cannot be set to zero in the DP. There is always a DRD, the vacuum energy. Thus lambda cannot be set to zero either. Even if there is no recognisable DRD with gravity in 3D (no fluctuation in spacetime between DRD and gravity), there must be a counter term for the vacuum energy. Then gravity and DRD are only the compensation for the fluctuation in spacetime. The cosmological constant is then a repulsion from positive energy. No DRD is actually needed. This is a property of spacetime itself. This is then a constant dilation without curvature away from a DRD. Thus an expansion. Elementary particles other than photons would only undergo this dilation for the vectorial part (momentum) of the DRD. The SL in 2D cannot be pulled apart by this. The redshift in the photon is then directly the expansion of spacetime. An SL would then have to become explicitly larger, since it is a geometric manifestation in the stretched spacetime.
Disadvantage: Then the Planck SL of the DM would also have to become larger. The term simply determines a property of spacetime without direct proof.