The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
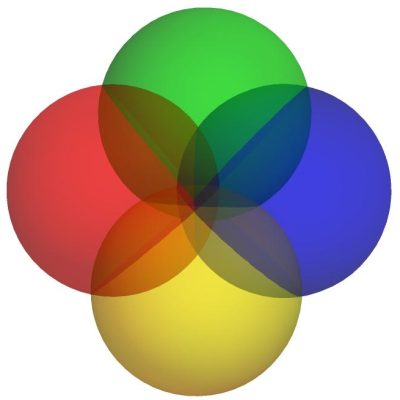
Binding energy as intersection of spacetime density.
6 Standard model (construction site)
leer
The structure of the Standard Model (SM) of particle physics is explained in a “fly-over”. All objects of the SM are geometric images of the 3D DRD in the different low-dimensional spacetimes. In 3D, only the Compton wavelength is known as a mapping of the DRD. This determines the content as energy or the density of the DRD itself. The curvature of space, intrinsic as well as extrinsic, is the only property that presents itself differently over the different geometries. The quantum fields of QFT are the various combinations or intersections of the lower dimensional spacetimes. An intereaction between objects can only exist if the objects have an expression of the respective geometry of the interaction.
QFT thus represents the low-dimensional DRD as “portions” in 3D and cannot describe continuous gravity in 3D. The low-dimensional geometry does not arise by itself in these spacetimes. The DRD is statically imposed by 3D on these spacetimes.
Difference between fermion and boson
The DRD also begins and ends with zero in the low-dimensional. Gravity is also generated in the low-dimensional spacetimes by the DRD. With bosons, the DRD is balanced directly in the object. Like a wave with full wavelength. The fermion corresponds to only half a wavelength. The DRD does not cancel itself out in the individual object. This property is mapped via the integer or half-integer spin. Since a DRD is always a state of motion, a rest mass is in itself a “scalar state of motion”. This is understood as a kind of torque that can be measured in all directions, the spin.
The 2D expansion plays no role in 3D because of the dimensional transition. Thus, the assumption of a point-like particle is as correct as it is wrong. Only the Compton wavelength corresponds to the extrinsic expression and is directly recognisable as a geometric quantity.
The three particle families of the fermions arise from the fact that the geometric expression of the first families can intersect orthogonally a maximum of 3 times in a 3D spacetime. The particle families become heavier and heavier through the connection of more space dimensions. The more space dimensions involved in a DRD, the harder it is to change a spacetime. Since these objects are composed of intersections of several spacetimes, they are not stable. With elementary particles, there are two possibilities.
- Bosons: If the spin is 1 and the DRDs cancel out, these objects cannot form intersections with other objects in spacetime. Thus, no particle families can form. A boson can be composed of intersections. However, it itself cannot form any more intersections.
- Fermions: If the spin is ½ and the DRD does not balance out, the expressions can influence each other over the possible 3D space dimensions and form particle families.
The Higgs boson as well as the neutrinos have a special role.
The different charges are the geometric alignments of properties in spacetime.
Electron, muon, tauon
The electron is the simplest particle in the DP. An BH in a 2D spacetime. For a pictorial idea of the geometry, the Flammer paraboloid is suitable. This shows an BH in a 2D version with intrinsic as well as extrinsic space curvature. The properties of an electron are as follows:
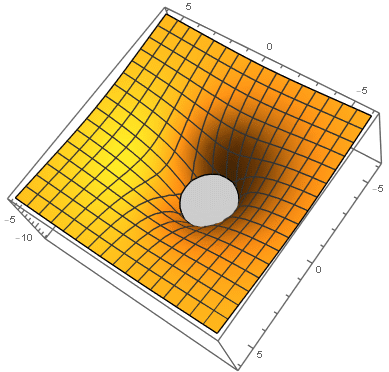
Mass: The mass is the number of spatial dimensions occupied together with the BH. The size of the BHS in 2D does not play a role here. Since all fermions have a rest mass, they must occupy at least 3 space dimensions. The mass is a resistance to reach the dimensional limit (SL). The more space dimensions involved, the heavier the DRD of the object can be increased. In the case of an electron, a 2D spacetime, with the dimensional transition from the singularity of the BH, has become 3D again. In the case of a muon, a second 2D spacetime with a separate BH is added. At least one space dimension of the two spacetimes must be identical. The second space dimension must be identical to the extrinsic deflection of the second BH. This means that the two 2D spacetimes are orthogonal to each other. In the case of a tauon, 3 surfaces and 3 BH. The jump in mass from electron to muon is larger, because here a new space dimension is added with the new surface. With the jump from muon to tauon, all spatial dimensions are already present. Therefore, this jump is not so big. The stronger “anchoring” by the BH is added.
With more than one BH, the new space dimension cannot remain orthogonal to the surfaces. The SLs in the different spacetimes have identical space dimensions. Therefore, the BHs must merge. This is not a stable state. Therefore, muon and tauon have a decay time. The more different spacetimes the shorter the decay time. An electron cannot have a decay time because its BH is a static image.
Here is a picture of the intersections for muon and tauon
Charge: The electric charge is described in more detail in interaction. Here it is only a question of why an electron always has the same elementary charge. An BH must also have a gravitational field and a deflection in 2D. Since there are infinitely many 2D spacetimes, other 2D spacetimes must intersect. All 2D spacetimes that have exactly one identical spacetime dimension must participate in this gravitation and also have a gravitational field. Even though the gravitational field here is a 2D field, our 3D spacetime is filled via the infinite number of 2D spacetimes.
The simplest way to imagine this is to give the 2D space point a 3D coordinate system. Through the BH, all 3 are again present. Here, on each axis, any set of 2D spacetimes can have a common spatial dimension and fan out around that axis. This works exactly 3 times in 3D. Therefore, the elementary charge of 1 is actually 3 * 1/3.
Muon and tauon have no higher charge than an electron, because all 3 space dimensions are already present in the electron.
Here picture of fanned out intersections
Spin: Spin is not a rotation in the classical sense. It corresponds to the extrinsic “deflection” in the space dimensions. Since the electron is an BH, this deflection is not balanced in the spacetime in which the BH lies. It corresponds to only half a wave. Hence a spin of ½. The interpretation as rotational motion comes from the scalar state of motion of the DRD in 3D. This is motion without a preferred direction. The same in all directions. The closest thing to this is a rotational motion in all directions simultaneously. Therefore, the spin can be measured to all possible axes. In the superposition, this must move in equal parts “up” and “down”.
For muon and tauon, there is no change in this view.
Quarks
The difference from the previous leptons to the quarks is that the SL is distributed directly over two or three 2D spacetimes. Not a composition as with muon or tauon. The BH itself is directly distributed on 2 or 3 surfaces. For d, s and b the BH is on 2 faces and for u, c and t it is on 3 faces simultaneously. Thus the DRD from 3D, above the image of an BH, can be distributed on 1 (electron, muon, tauon), 2 (down, strange, bottom) or 3 (up, charm, top) surfaces. More possibilities are not available in 3D for 2D.
The d-quark is the simplest case. Think of the geometry as an angle. The BH has 2 planes combined into one object. But the BH cannot be “crossed” in both planes. It can only be half on one plane each. The two planes have a 1D intersection. The d quark is not a muon! There are not 2 complete BHs intersected. Here, the one BH is divided into 2 planes. Therefore, the properties of the BH are in the 1D intersection. A single quark can thus not appear in 3D. There is no additional spatial dimension due to a single quark. Not even all 3D space dimensions are fully occupied.
Here image of angle
Mass: There are 2 surfaces connected to an BH. Thus the mass must be greater than for electron and less than for muon.
Charge: The property of the BH lies in the 1D intersection. Therefore, only those 2D spacetimes can connect to the BH that have a common space vector in the 1D intersection. They are only 1/3 of the spacetimes as in the case of leptons.
Spin: The spin remains at ½ for d, s and b because there is no compensation on the plane.
In the case of the s quark, one part of each of the 2 BHs lies together in one plane.
Here picture of 2 angles with overlap in height. Not a complete cross as with the muon
Mass: 2×2 surfaces are connected with 2 BHs. Whereby 2 surfaces are identical in each case. This means that the mass is greater than that of the d quark. The structure is close to the muon, but not identical. Therefore, the s-quark has a very similar mass to the muon. Since a part of the “cross” is missing, the mass must be somewhat lower.
The b-quark is two s-quarks, these now cover the cross completely with 4 BHs.
Here is a picture of the cross with 4 BHsMasse
There are 2 surfaces connected with 4 BHs. This means that the mass is greater than that of the s-quark. Since there are 4 BHs, the mass is higher than that of the tauon.
The u quark already occupies 3 surfaces.
Picture 2 Wrap where the bottom surfaces overlap but the height is at a 90 degree angle.
Mass: There is a deviation here. The mass is lower than that of the d quark, although 3 surfaces are already involved. The difference is the area where the BHs overlap. This area is not sufficient for the actual geometry! Therefore the area of the overlap must be smaller and the mass falls below the mass of the d-quark.
Charge: The charge is now 2/3, since there is 2 times a 1D overlap.
Spin: Remains the same for all quarks at ½.
c-quark is like u only 180 degrees to the right.
The t-quark must have the expression of the c-quark 4 times in order to occupy the complete geometry. Therefore, it is also the hardest to divide. The two 1D intersections, are extended in length. The t quark, occupies all spatial dimensions and therefore cannot combine with any other quark.
4x c quark, to occupy all the spaces
Neutrino
Like all fermions, the neutrino is divided into 3 different particles of the families. These can then transform into each other. In the DP, the neutrinos are constructed somewhat differently. The neutrino has a special position among the fermions. The neutrino forms from a 1D spacetime and is thus subject to different conditions than the other fermions.
- In 1D, there can be no intrinsic space curvature. The space curvature in 1D is only extrinsic. This is sufficient in 3D or 2D to produce a higher DRD. Neutrinos cannot participate in electromagnetic WW because they have no surface. In 1D, no SL can form.
- Since a transition from 3D directly to 1D is not possible due to the limits of spacetime, the neutrino always appears in combination with other fermions. A pure neutrino reaction cannot exist. Not even with neutrino and anti-neutrino. Therefore, neutrinoless double beta decay must not exist. All recognisable properties of a neutrino must be above 1D at least in 2D. Therefore, they appear like leptons.
- For 1D to be detectable and have mass, a neutrino must occupy all 3 spatial dimensions via a 2D mapping.
- There is only one neutrino that transforms into all 3 versions based on spin and state of motion. Actually, it does not transform. It changes appearance via occupying 2D.
- Since it is only 1D with a 3D appearance, the neutrino has by far the smallest rest mass.
- As shown with bosons, the weak nuclear force is a mixture of 1D and 2D. Therefore, a neutrino can only participate in this interaction, for DRD exchange.
Bosons and interaction
Gravity falls out of this consideration. This is the curvature of space in 3D without an exchange particle. Gravity changes the definition of space directly in 3D and does not have a different lower-dimensional image. Thus also no exchange particle. All other fundamental forces have a lower dimensional geometry and for this geometry an exchange particle.
It is a much simpler assumption that all quantum fields should be a combination of low-dimensional spacetimes than to have a separate field for each particle and each fundamental force. The transformation of the types of particles and matter and energy is then much more natural than with various different fields.
Since bosons are self-equilibrating in spacetime, there can be any number of bosons at one point in space from a 3D point of view. If the spin is 1/2, spacetime is not balanced and the fermions are subject to the Pauli principle.
Electromagnetic interaction
The electric field and the magnetic field are directly the gravitational fields in 2D. The difference in the fields is that the electric field is the original gravitational field in 2D. The magnetic field is created by the movement of the electric field. Through the movement of the electric field, other 2D universes are “pulled along/pushed through”. These are then the magnetic field and can never have a source for the field and must be divergence free. Therefore, there can be no magnetic monopole.
The electric field is created by a particle with an BH. Then there is always a source. The similarity of gravity and electric field is thus obvious. Electromagnetic interaction, like gravity itself, must therefore have no limit in range.
The exchange particle photon is a gravitational fluctuation in 2D. Thus more space comes together in a volume and the fluctuation corresponds to a DRD. A photon does not change its representation as a wave without an interaction. The gravitational fluctuation is explicitly static in 2D. According to the GRA, there are not enough degrees of freedom for dynamics in 2D.
Since an electric field is a 2D gravitational field, only a 2D gravitational fluctuation can produce an interaction between the objects. Without a 2D component in the geometry (surface), one never participates in the interaction.
The intrinsic space curvature is always bound to an extrinsic space curvature. From 2D, this results in a positive and a negative expression. These can cancel each other out. However, the DRD cannot simply disappear. Therefore, it must remain as a pure fluctuation without a source. Since spacetime wants to balance itself out, charges of the same name repel each other and different charges attract each other. Every 2D-BH has already reached the limit of spacetime in 2D. If another 2D-BH with the same deflection is now added, the boundary cannot “expand” again within 2D. If an opposite interpretation is added, the DRD can be mapped away from the boundary back into 2D spacetime as a fluctuation.
Weak interaction
Is an interaction that uses 2D and 1D at the same time. Thus all other particles participate in this interaction. Since the interaction consists of intersections of 2D, this can be united with the electromagnetic interaction. All bosons of the Weak interaction have BHs themselves and must fully occupy the geometry. Are not a full wave and still spin 1, because the overlap on the space dimensions closes again via the geometry. Same state as wave. Short range due to enormous mass, as linked in all dimensions. The vectorial DRD lies opposite in the space dimensions, also therefore no range.
Z-boson is electrically neutral, since the 1D intersections cancel each other out. Is particle and anti-particle in one. Has the shape of a cube.
The W boson is a star that occupies all space dimensions and thus has a full charge.
Strong interaction
Is a wave that imprints itself on 2 different planes. 1 time in the horizontal plane and once on the vertical plane. Both wave planes have a bend of 90° to each other. Thus no clear vectorial DRD and no range. The wave itself is balanced, hence spin 1. However, there is only half a wave in each plane. Therefore the gluon as exchange particle is itself carrier of the charge. Always positive and negative in different charges.
There are several versions of gluons. However, these do not represent a family as in the case of fermions. The gluons themselves are not composite objects. There are several ways in which a wave can split into 2 levels.
The confinement results from the geometry with a corner/winding in the case of the quarks and gluons. One quark alone does not completely occupy the 3D space. Therefore, there must always be at least two quarks. These are connected by the gluons. If you now want to remove two quarks from each other, you have to “tear” the gluon. To do this, you have to put so much energy (DRD) into it that you can create new quarks.
Higgs field
The Higgs field is the maximum combination of the low-dimensional spacetimes in 3D. If the t-quark did not have so many BHs, the Higgs boson would be the heaviest particle.
The H boson is the only particle that is directly a 3D particle. Therefore it does not participate in the other interaction and cannot have a spin. The only property it can have is mass.