The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
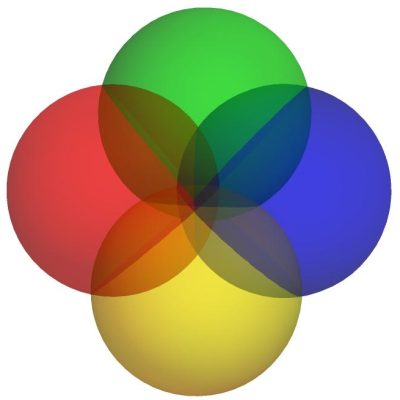
Binding energy as intersection of spacetime density.
Planck-Länge
leer
It is calculated how far 2 electrons can approach each other. Two boundary conditions are set:
- The largest possible force between the electrons is the reciprocal of the proportionality constant from Einstein’s field equation \frac{c^4}{8\space *\space \pi\space *\space G} the Planck force.
- Since the 2 electrons should be very close, the fine structure constant α must be used. The force between the electrons is exchanged via a photon and a consideration from a quantum mechanical point of view must be chosen.
Step one is the comparison of the force from the field equation and the force between 2 electrons
\cfrac{c^4}{8\space *\space \pi\space *\space G}\space =\space \cfrac{e^2}{4\space *\space \pi\space *\space \epsilon_0\space *\space r^2}
Step two is the fine structure constant because of the exchange of a photon. The largest possible force must be reduced by this value.
\cfrac{c^4}{8\space *\space \pi\space *\space G}\space *\space \cfrac{e^2}{4\space *\space \pi\space *\space \epsilon_0\space *\space c\space *\space \hbar}\space =\space \cfrac{e^2}{4\space *\space \pi\space *\space \epsilon_0\space *\space r^2}
Step three is shorten everything and resolve by distance r.
\cfrac{c^4}{8\space *\space \pi\space *\space G}\space *\space \cfrac{1}{c\space *\space \hbar}\space =\space \cfrac{1}{r^2}\space \iff\space r^2\space =\space 4\space *\space \cfrac{h\space *\space G}{c^3}\space \iff\space r\space =\space 2\space *\space l_P
Electrons can approach up to two Planck lengths. Then the force would rise above the maximum. If a photon is to be exchanged, then the wavelength must be larger than the Planck length. Otherwise the photon is a BH. Thus the distance must be larger than a Planck length.
The same result is obtained if again the largest possible force is multiplied by an unknown length to obtain an energy. This expression is compared with E\space =\space h\space *\space \nu. Where the wavelength in ν must be the same as the length in the force. From this follows:
\cfrac{c^4}{8\space *\space \pi\space *\space G}\space *\space r\space =\space h\space *\space \cfrac{c}{r}\space \iff\space r^2\space =\space 4\space *\space \cfrac{\hbar\space *\space G}{c^3}
The difference is that h in the result, must be the reduced quantum of action. It follows that the largest force can be \frac{c^4}{8\space *\space \pi\space *\space G} or \frac{c^4}{4\space *\space G} Depending on whether one chooses the reduced view or not.
Also in the Bekenstein limit, the smallest footprint is calculated to be 2\space *\space 2l_P.
It seems that in our spacetime a geometrical expression must always be assumed with at least two Planck lengths.