The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
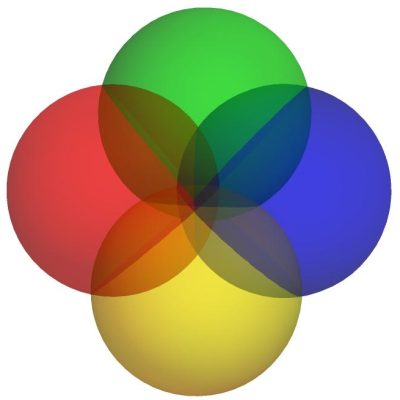
Binding energy as intersection of spacetime density.
3 Spacetime structure
leer
Since in the DP everything consists directly of spacetime, it is useful to have a closer look at the structure of spacetime. In particular the natural constants c of SL and G of gravity are the central quantities here. A transition of different dimensional spacetimes is described. This is needed later for the QFT. For the investigation of the spacetime three aspects are considered: the dimensional boundaries, a smallest element and an absence of the spacetime.
There are innumerable treatises how big our universe can be and which borders it has. All these considerations have in common that these limits are defined by a concept of distance. This is the natural procedure for objects from the everyday life. For spacetime, this notion is not helpful. The spacetime defines the geometry with which one wants to describe the spacetime (distance). Outside of spacetime, no geometry is defined. Therefore, spacetime as a separate object is a structure that has no boundary in the sense of a distance to something else (embedded). That is why the number of spacetime dimensions is used as a boundary of spacetime. The following cases are considered as dimensional boundaries. Our 3D spacetime with one spacedimension less and one spacedimension more.
Speed of light c
If a DRD has the state of motion of the SL, then a space dimension and the time dimension are missing. According to the SR both dimensions are explicitly zero. From the point of view of the DP, one can use the result as a definition. The SL is exactly the state of motion where the mapping of the DRD has only two space dimensions. Thus, the natural constant c represents the low-dimensional limit in our spacetime. It becomes clear why the sL is this extreme limit for any DRD in spacetime. All DRDs are spacetime and this has a boundary at the SL itself.
If a space dimension is missing, then also the time dimension must be gone. Our spacetime has one spacedimension less at the SL. If the time is bound to the space, then this must become zero together with the space dimension. To have one spacetime dimension less and the identical time dimension as the spacetime in which the spacetime dimension was taken away is not possible. A photon, which moves with SL, cannot recognize the 3D-space and the time in our spacetime. A photon has not disappeared from our spacetime. Thus, SL represents the lowest limit at which one can still perceive a DRD. It follows that there can be no transition from 3D directly to 1D.
The state of motion of the SL is the low dimensional boundary
For the low-dimensional limit, c is the appropriate natural constant. This can be given as a equation in two different notations.
About the Planck length and Planck time: c\space =\space \frac{l_P}{t_P} with l_P as Planck length and t_P as Planck time. Since the denominator and numerator of the fraction can have an arbitrary combination to represent c, there must be at least one more condition for the Planck sizes. The fraction says that you cannot get a certain length of space dimension closer to the dimensional limit (time). In other words, where there is a length, there must always be a minimum amount of time. From this follows that the length and time of zero within the spacetime do not make sense. There must be a transition in a low-dimensional spacetime.
About the natural constant of the electromagnetic intgeraction: c^2\space =\space \frac{1}{\epsilon_0\space *\space \mu_0} with \epsilon_0 as electric field constant and \mu_0 as magnetic field constant. These values seem to have nothing to do with the first formula. By the dimensional transition one can already recognize that these nature constants are bound to a low-dimensional expression. For a representation of 2D in 3D, however, a basic quantity of space and time is needed. This is given by c. More about the electromagnetic intgeraction in the chapter Standard model.
Gravitational constant G
If there is a lower-dimensional limit, then there must be also a higher-dimensional limit. With the length contraction to zero by the SL, one has used an extreme event in the spacetime for the low-dimensional boundary. For the higher-dimensional limit, the only other extreme event in the spacetime is used, the singularity from the GR.
Explicitly not the event horizon (EH) of a BH. This does not represent a special boundary in the DP. The EH is explained in the following chapter “GR with DRD”.
For the singularity one can choose two different views. The mathematical and the physical view.
- Mathematically: From a purely mathematical point of view, a spacetime can be stretched to infinity. The singularity can be described as follows. On a volume of zero (r = 0), an infinite curvature of space is contained. This point acquires a new spatial dimension due to the curvature. Since the dilation is infinite, there is actually no spacetime contained. This is a very good description for the transition into a higher-dimensional spacetime. From the higher-dimensional point of view, all the properties of lower-dimensional spacetime, in a volume of zero, are contained. From a physical point of view, the spacetime region with r = 0 cannot be reached by gravity in its own spacetime.
- Physical: A mathematical singularity cannot arise. This has the following reasons:
- An BH does not receive an infinite amount of DRD. Since gravity is generated by DRD, it is never infinite.
- According to the low-dimensional limit, there cannot be a point with zero extension in all space dimensions, otherwise this point in space and thus the singularity would no longer exist in spacetime. The gravitational effect would have to disappear in the singularity. However, gravity keeps adding up and does not decrease. The DRD is not cancelled out by gravity. Gravity is generated by the DRD.
- The DRD is a density and thus always requires a volume. Gravity can only create strain up to the boundary of the DRD. Not within the DRD. Thus gravity cannot reach the point r = 0. If gravity were within the DRD, it would have to cancel out the DRD. Again: Gravity in an SL does not disappear.
- Since the DRD is a density, it can overlap in the core of an SL in the same volume (more on this later). There is no “space problem” there. Any amount of DRD fits in the centre of an SL.
In contrast to SL, one loses nothing here and one remains in one’s own spacetime. The time dimension does not have to become zero locally. Even if no mathematical singularity is physically reached, this singularity represents a limit. To avoid confusion, the term singularity is still used for the centre of an BH
The singularity in the BH is the higher-dimensional boundary
This higher-dimensional limit, like the lower-dimensional limit, is assigned a natural constant: G is the gravitational constant. As with c, a statement must also be made with G about the sizes of spacetime. G must describe how a spacetime behaves when a DRD is present. It is best to start with the textbook definition of G.
G\space =\space \cfrac{l_P^2\space *\space c^3}{h} This form is not suitable for consideration within the DP. All values of the Planck scale are not considered reduced in the DP.
We rearrange the equation. A Planck time is extracted from the h and united with a Planck length from the numerator to form a c.
G\space =\space \cfrac{l_P^2\space *\space c^3}{h}\space \iff\space \cfrac{l_P\space *\space l_P\space *\space c^3}{E\space *\space t_P}\space \iff\space \cfrac{l_P}{E}\space *\space c^4From this conversion, 3 versions are generated, which in principle all make the same statement:
Version 1: \cfrac{l_P}{E}\space *\space c^4
G now consists of 2 terms.
- c^4 : Since we want to describe G in a spacetime with 3 spacetime dimensions and 1 time dimension, we need the low-dimensional boundary exactly 4 times.
- \frac{l_P}{E}: This term indicates what happens to a length in which a DRD (energy) lies. We call this term the “dimensional constant (DC)”. This will be expanded again later. This DC is the actual counterpart to c. The energy corresponds to the energy content of a Planck mass. If we put the DRD of a Planck mass on a Planck length, we get an BH and thus a singularity. Since we have defined the force as a change in a DRD, it is clear that a reciprocal force must appear as a counterpart in gravity. The DC is the reciprocal Planck force.
G describes exactly, the behaviour of spacetime between the two boundaries.
Version 2: \cfrac{l_P}{m_P}\space *\space c^2
Here, the same facts are described only with mass instead of energy. Since a mass is a low-dimensional image, c may only be counted for the two spacetime dimensions with which our spacetime is then connected. Explanation of the dimensional transition comes later in this chapter.
Version 3: \cfrac{l_P}{m_P}\space *\space \cfrac{1}{\mu_0\space *\space \epsilon_0}
As has been established with the natural constant c, one can also write a c^2 in this form. The second term is a kind of “spacetime resistance from low-dimensional spacetimes”. More on this later with the electromagnetic intgeraction.
Now one can investigate the proportionality constant k in the field equation with the DC.
k\space =\space \cfrac{8\space *\space \pi\space *\space G}{c^4}\space \iff\space \cfrac{8\space *\space \pi\space *\space \cfrac{l_P}{E}\space *\space c^4}{c^4}\space \iff\space 8\space *\space \pi\space *\space \cfrac{l_P}{E} The value is 8\space *\space \pi\space *\space 8,267\space *\space 10^{-45}\space [\cfrac{1}{N}]
The DC is sufficient for the field equation. The tensors (unlike G) are already designed for 3D spacetime and do not need any more additional c. The DC is a resistance of spacetime against a stretching. A DRD produces only a very small gravitation. As will be shown later, this has to do with the fact that a DRD is always a low-dimensional image. 2D is almost unrecognisable in 3D.
No quantisation in 3D
In the previous view of spacetime, there is no form of quantisation. This applies to spacetime itself and to gravity as well as to DRD.
DC is a reduction of change, but it is not a quantisation. In spacetime there is no reason for quantisation. Spacetime and all the mappings in it are continuous.
Why we observe quantisation in DRD is described in the chapter “Quanta and Waves”.
Transition between 3D and 2D
For the QFT, a transition from 3D to 2D is still needed. The boundary itself has already been described. For this boundary, an important question remains open: What can be transferred across this boundary? To anticipate, virtually nothing. Let’s take a closer look at the facts.
An object in a 2D spacetime has no volume and no surface in a 3D spacetime and thus no extension. In 3D, mathematical properties such as length, width or area can be attributed to a 2D object. Without an extension in its own spacetime, no geometric property can be determined. Properties of lower-dimensional objects in spacetime can thus not be transferred across this boundary. This is generally true for all geometric quantities. All objects are a DRD and a DRD is a density. A density requires volume.
The transition can only be made from properties that are directly contained in the spatial dimensions. These spatial dimensions must be identical. Only an extrinsic and/or an intrinsic space curvature or a DRD can be considered. Then these properties can be determined via the identity of the space dimensions. This also means that a 3D DRD must influence a 2D spacetime and vice versa.
To go from 3D to 2D, one spatial dimension must be set to zero. This does not happen in the first example as in all physics textbooks, please. To simplify the example (which is correct) only one space dimension is considered and the rest is set to zero. As described in the DP, there is only one possibility to physically set a space dimension to zero, the SL. This means, however, that the time dimension is also always zero. Time is bound to the given space.
As a result, the intersection of a 3D spacetime and a 2D spacetime consists of only two spatial dimensions. Time is unique in each spacetime and cannot be transferred.
Since the space dimension in only one direction can be set to zero by the LG, there cannot be a “true zero of all space dimensions” in a spacetime. The geometric quantity zero in a spacetime on more than one spacetime dimension is excluded, there can only be the SL as a low-dimensional limit.
If a 3D DRD has a connection to a 2D spacetime, via the spacetime dimensions, then this 2D spacetime must also have a DRD with identical extension. This DRD, and thus the extension, only comes via the connection and is not itself a property that is generated in 2D. All properties are “imposed” from 3D to 2D. All additional properties through the 2D-DRD mappings must be in the range of the 3D-DRD, but have no extension themselves. The view that an elementary particle has an extension (in 3D), but the properties are point-like, sounds strange at first, but is completely correct.
Time as distance to the lower dimensional boundary
From these considerations, one can derive a different interpretation for time. In the DP, time is seen as a distance measure to the dimensional limit. If one approaches the dimensional limit, time passes more and more slowly. If one moves away from this boundary, time passes faster. You do not leave spacetime. Therefore, time continues to pass in an BH until the singularity. Since in our universe every object consists of DRD and DRD directly represents a state of motion, time passes for every object unless it moves with the SL.
A direction of the state of motion plays no role in this consideration, since the dimensional limit is the same for all points in space from any direction.
Time changes from classical physics to relativistic physics. From a rigid entity to a dynamic entity that forms a unit with space. In almost all considerations, however, time is still a global time for all dimensional considerations. In DP, every possible spacetime is now assigned its own time. Only space dimensions can connect across the dimensional boundary. Time is completely separated in different spacetimes.
If a DRD exists in a spacetime, then it is directly on the boundary and no time can be determined or it is between the boundaries and thus always has a distance to the boundary. Therefore, a DRD with rest mass always has time. If a DRD were to interact with “nothing”, then time as such would exist, but no time flow would be detected. Since DRD is constantly interacting (in the end only with the vacuum) the flow of time for a DRD between the boundaries is always present. The time flow is thus simply the sequence of the change of DRD through interactions. Therefore, in the mathematical description, this can go into the future and into the past. A change can also be calculated backwards. The change itself is the flow of time and thus not reversible for us.
The dynamics of time from spatial point in time to spatial point in time cannot be determined locally for a DRD and is therefore always the same. We cannot determine any difference in distance. This is only possible in a comparison with another DRD.
Vacuum energy
In the DP it has been established that the DRD is an identity to the energy. The density itself cannot fall to zero, otherwise there is no more spacetime at this point and the spacetime under consideration does not exist. Thus, based on the definition of DRD, an energy of zero at any point in spacetime is ruled out. Where there is spacetime, there is also energy. From the considerations of the spacetime boundary, a DRD with a volume of zero can also be excluded. Thus, in spacetime, the existence of any spacetime within a volume is always given.
Information and spacetime
For an understanding of QFT, one property of information is still missing. This is always bound to the spacetime in which the information is present.
A property of a single object is not yet information. No statement can be made about the property. Only when this property is known at at least one other point in spacetime has information come into being. Information refers to the knowledge of at least one property at a different point in spacetime than the object itself. This means that information is always bound to a distance in spacetime and is therefore a 3D object.
Information occupies spacetime
It follows that in the case of an interaction of many objects, information is formed in spacetime about the object as a whole. The individual object is only partially determined by this information. The existence of information is thus not necessarily binary. A piece of information can become more and more “anchored” in spacetime via many DRDs with interactions.
The connection of the information with spacetime is later in QFT the main reason why no information can be transferred in the case of entanglement. Also the double-slit experiment with the path information, especially in the “delay choice” variant, becomes relatively simple with the different time in the spacetimes and the information with spacetime.