The speed of light is the low-dimensional limit
The singularity in a black hole is the higher-dimensional limit
With r_S\space =\space \frac{2\space *\space l_P^2}{\lambda}, the Schwarzschild radius is directly related to the Compton wavelength
leer
Dimensional Physics
leer
Theory unifying general relativity with quantum field theories
Christian Kosmak, Germany Würzburg 2023 Version 4.1 – 05.30.2023
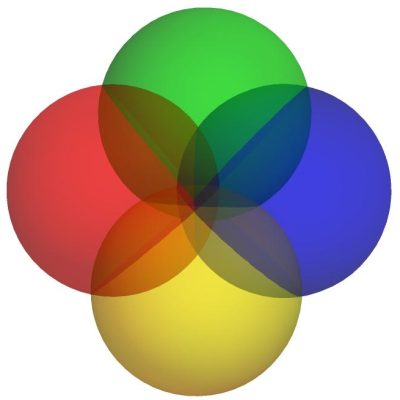
Binding energy as intersection of spacetime density.
5 Waves and quanta
leer
It is described how a quantisation and a wave description arise. The dimensional transition is needed for this. The “oddities” in QFT almost all come from the dimensional transition without time. For a first approach, start at a different place and extend DC. The gravitational constant G is to be built up more generally in the formula with the DC. This then results in a connection that generates the dimensional transition.
Dimensional constant (DC) complete
The DC describes quite generally the possible change/resistance within spacetime. Therefore, it must also provide a description for DRDs that do not correspond to the Planck mass. In the previous chapter, the DC in G is derived from the description G\space =\space \frac{l_P}{E}\space *\space c^4.
Here E stands for the energy of the Planck mass. Our universe is filled with DRD, which has much lower energies. Therefore, the term of the DC must be extended by a correction. A term is needed that has no effect with the Planck mass.
First, the definition of the Compton wavelength is expressed differently. As with G, we rearrange the formula until a clear statement is included. We start again with the textbook definition. It decomposes h into energy times Planck time. The energy becomes Planck mass times the square of the SL. One SL cuts out. The remaining SL is decomposed into Planck length by Planck time.
\lambda_C\space =\space \cfrac{h}{m_C\space *\space c}\space \iff\space \cfrac{m_P\space *\space c^2\space *\space t_P}{m_C\space *\space c}\space \iff\space \cfrac{m_P\space *\space c\space *\space t_P}{m_C}\space \iff\space \cfrac{m_P\space *\space l_P\space *\space t_P}{m_C\space *\space t_P}\space \iff\space \cfrac{m_P}{m_C}\space *\space l_Pm_C\space *\space \lambda_C\space =\space m_P\space *\space l_PThe Compton wavelength is simply the Planck length multiplied by a mass ratio. The Compton wavelength must be directly related to the Planck length.
The relationship found is inserted into the modified formula for G. Here, the energy of the Planck mass is replaced by m_P\space *\space c^2. Then the Planck mass by the expression just found.
G\space =\space \cfrac{l_P}{c^2\space *\space m_C}\space *\space \cfrac{l_P}{\lambda_C}\space *\space c^4\space \iff\space \cfrac{l_P}{m _C}\space *\space \cfrac{l_P}{\lambda_C}\space *\space c^2Now any DRD can be specified for the mass. The Compton wavelength corresponding to the mass must be used. In the case of the Planck mass, this is the Planck length. Thus the second term has no effect. The DC indicates that a wavelength is required for all masses that do not lead to an BH. This wavelength corresponds directly to the Planck length and the mass itself. The smaller the mass, the larger the wavelength. This is the first indication that a wave representation is absolutely necessary.
Does the DC also result in quantisation? Explicitly no! To show this, G is used in the given form in the calculation for an SSR. M must be equated with m_C.
r_S\space =\space \cfrac{2\space *\space M\space *\space G}{c^2}\space \iff\space \cfrac{2\space *\space M}{c^2}\space *\space \cfrac{l_P}{c^2\space *\space m_C}\space *\space \cfrac{l_P}{\lambda_C}\space *\space c^4\space \iff\space \cfrac{2\space *\space l_P^2}{\lambda_C}\bold{r_S\space =\space \cfrac{2\space *\space l_P^2}{\lambda_C}} This little formula is important for the DP!
Three important statements emerge:
- In the formula : r_S\space =\space \frac{2\space *\space l_P^2}{\lambda_C} the wavelength λ is replaced by the wavelength for an BH, the Planck length. Then this gives r_S\space =\space 2\space *\space l_P. The smallest possible BH that forms in our spacetime has an SSR of at least two Planck lengths.
- Solved for the Planck length, l_P\space =\space \sqrt{\frac{r_S\space *\space \lambda_C}{2}} results. Since the Planck length is a constant, neither the SSR (from GR) nor the Compton wavelength (from QFT) can become zero or infinite. There is no quantisation for either quantity. Via the Planck length, one obtains a correlation for the representation of a DRD and gravity. Thus gravity and DRD in their different representations are mutually bound to this limit.
- r_S\space =\space 2\space *\space l_Pis the smallest possible BH in 3D. For the rest mass of an electron, with the appropriate Compton wavelength, this results in an SSR of about 10^{-57} metres. This SSR cannot have formed in 3D. This is true for all Standard Model objects. If the equation is correct and there is an SSR for every Compton wavelength, then this SSR must have formed in a spacetime that has a different Planck scale. The hierarchy problem is the solution here. A spacetime is needed where an SSR can form with a smaller DRD at a longer length. A 2D spacetime. A 2D spacetime as a surface is much easier to change (DC) than a 3D spacetime with volume.
The SSR from ART and the Compton wavelength from QFT can be mutually exchanged. Both values are not quantised. The Compton wavelength replaces the mass and the gravitational constant together for the calculation of the SSR. From this, one can conclude that the Compton wavelength directly indicates the change in spacetime due to the DRD as a wave representation.
Low-dimensional representation of the DRD
The DRD in 3D does not have a specific geometric shape. This is simply a density. Even if it had a shape, we would not be able to recognise it. A metre remains a metre. This is true not only for the length, but also for the geometric shape. Here, the last assumption must now be made so that anything at all can be recognised.
Assumption A-03: There are infinitely many separate low-dimensional spacetimes.
Our spacetime was created by a big bang. Why then not other spacetimes with different numbers of space dimensions? The Copernican principle is generally applied to spacetime. Our spacetime is therefore not special. An infinite number of lower-dimensional spacetimes fit into our spacetime. The concept of quantising spacetime is applied here, in a completely different form, as the concept of separating spacetime. Not to the content of a spacetime, but to the spacetimes as individual objects. Each spacetime is a separate object in itself.
Thus, within a DRD there is an infinite set of low-dimensional spacetimes. As stated in the chapter on spacetime structure, these can be connected to each other via the spacetime dimensions. It follows necessarily from this that the lower-dimensional spacetimes must react to the change in the spacetime definition. There are basically two different ways in which these low-dimensional spacetimes can react to the DRD, with and without rest mass.
Wave representation without rest mass
As an example from the Standard Model, we use the photon here. It is the simplest particle for this representation in the DP. The DRD in 3D can simply be imagined geometrically as a sphere (volume) in which the spacetime has a definition that corresponds to a higher density. Let us stay with one metre. The sphere has a diameter of one metre. Since the density is higher, we assume that the diameter contains a total length of two metres. If a 2D spacetime now lies in these volumes, it must accommodate two metres in a length of one metre without having its own DRD in 2D. The 2D spacetime will form a transverse wave. Longitudinal is not possible, as this would again represent a DRD in 2D spacetime.
Several properties are discussed, which then also apply to a wave representation with rest mass. There is only the difference for a wave representation with rest mass. This wave must now have the following properties:
- Boundary in the volume: Since the DRD has an expansion, the 2D wave must also have a boundary from which it is flat again. Another point is added to the “boundary”.
- Extrinsic and intrinsic: For an extrinsic wave to become flat again at the boundary, there must always be an intrinsic component. The proportion from extrinsic to intrinsic is directly connected. This intrinsic part is a 2D gravity and the fluctuation is a fluctuation of the electric field. In the Standard Model, an electric field is shown to be nothing more than a 2D gravity imposed by 3D.
- Equalisation: The wave representation equalises itself directly in 2D spacetime. This has two effects:
- The photon is not a source for the electric field (2D gravity). It is only a fluctuation. This fluctuation of 2D gravity has nothing to do with a gravitational wave in 3D.
- The wave is complete. There is always a wave-valley for a wave-mountain. The geometric representation in 2D is balanced in total. This property is later called spin. This is not only about the intrinsic part, but about the general interpretation of the wave from the plane.
- Static: The wave has an intrinsic component that can be directly regarded as gravity. According to GR, gravity in 2D has too few degrees of freedom for it to change. This again has two effects:
- The curvature of space in 2D cannot form on its own. It must always be “imposed” on 2D from 3D.
- The curvature of space is static because it cannot change. This means that the extrinsic as well as the intrinsic part of the space curvature is static. An expression in 2D remains as it was created. This applies to all expressions. This absence of the degree of freedom is identical to the conservation of energy. No change in time for any geometric expression. Only one transformation is possible. Then the geometric structure must dissolve and be redesigned. In this process, the 3D-DRD serves as a collection pot for all expressions. The view of QFT that the objects destroy and reform during an interactions is absolutely correct.
- Energy level: The 2D spacetime does not have to fully intersect the sphere of the DRD in 3D. It could also lie only partially in the volume of the DRD. Then a smaller wave must form in its expansion and amplitude. The proportion of DRD on the 2D spacetime is smaller. Thus a DRD, as a geometric manifestation in a 2D wave, has the possibility of possessing the entire energy spectrum.
- Possibilities: As can already be seen with the energy level, there are infinite possibilities in size and energy for the representation of the 2D wave. The 2D wave also has the possibility to appear in the entire volume of the DRD. All 2D possibilities can be pronounced at the same time. In 2D, the time from 3D does not exist. Thus there is no temporal overlap for the representations. Only with interaction would the DRD have infinite energy due to the 2D expressions. This describes the renormalisation in QFT. The mathematical infinity for all interactions at the same time is in fact not relevant. Only one interaction combines the 2D possibility in time with the 3D DRD. This part is then the observation. However, all possibilities in 2D can influence each other as a sum in 3D. These are all actually present.
- The DRD specifies the total amount of energy for the possibilities. These possibilities are all in 2D. The possibilities (virtual particles) have no effect on gravity in 3D, only the DRD in 3D itself has that.
- The path from the possibilities to the single expression is described in the section “Superposition, probability and collapse”.
- Limitation in spacetime: According to QFT, a wave may occur in the entire universe and not only in the volume of the DRD. This is absolutely correct. Since the DRD is a density of spacetime itself and there cannot be a spacetime with zero energy, the wave has the possibility of using all of spacetime as its location. A DRD has no boundary with an absolute zero energy except the boundary of spacetime itself. Therefore, the entire spacetime is allowed, not just the volume of the DRD. The probability of an interaction outside the 3D DRD volume is just very small. Thus, the 2D DRD theoretically has the entire spacetime available, even if the DRD is clearly localised for gravity in 3D. The question of how the spatially not 100% determined behaviour and gravity fit together is thus solved. The problem does not exist in the DP.
- Wavelength for the energy: The wavelength is sufficient to describe the energy content of a DRD. This was also used for the DC. Why not the amplitude? A wavelength always indicates the energy in a DRD. If you increase the amplitude, the volume automatically increases. This does not result in higher energy. If the energy content of a DRD is to be compared, the volume must be kept constant. Then the amount of 2D spacetime, of a wave in the volume, is only determined by the wavelength. More spacetime in a volume is a higher DRD.
- Amplitude for probability: If one wants to generate a WW in the volume of a DRD, one must actually (physically) meet an expression of the possibilities. Since 2D always meets 2D, it is not the volume but the area that is decisive. The 2D plane from which the amplitude emerges is irrelevant. Only the deflection of the wave is the expression of the DRD and is relevant. The deflection of the wave can always be understood geometrically as a circle. The area of the circle is for every wave. The circle number π is the same for all possible manifestations. Only the radius differs. Therefore, the square of the amplitude is the only important quantity for the “hit probability”. Since they are waves (mountain and valley), the wave with the largest area is not always hit. Even waves with a small amplitude can be hit.
Since there are only the photon and the gluon without mass in the standard model, here is a short advance on the standard model. With the gluon, the interpretation of a wave lies on 2 different 2D spacetimes. It has the spin 1, since everything balances out across the 2D spacetimes from a 3D point of view. But at the same time it is the carrier of the charge, since only a part of the wave lies in the respective 2D spacetime. Thus a gluon always has a positive and a negative charge. Although it has no rest mass, its range is very small. The wave lies on two different planes and has no clear direction for the momentum. A gluon cannot get out of the superposition of the DRD, which creates the quarks in the atomic nucleus.
Wave representation with rest mass
The second way in which a DRD is transferred to 2D is that a DRD is directly imposed in 2D spacetime. In this illustration, one must be careful with which values are calculated. The Planck scale in 2D is a different one. Until now, the DC was designed for our 3D spacetime. In 2D, these values must be different. Because of the hierarchy problem, it is clear that a 2D spacetime can be compressed or stretched much more easily than a 3D spacetime. For a greater length, a smaller energy must trigger the higher-dimensional transition. In 2D, a very small mass creates a BH with large SSR (relative to the Planck scale in 3D).
Across the dimensional limit, only the SL and the Compton wavelength remain as quantities. The SL is defined by the compression of a single spatial dimension. This is identical in 3D to 2D. The Compton wavelength comes from the connection of the spatial dimensions and must have the same length. All other dimensions are different. Therefore, one cannot simply “calculate into” from 3D to 2D.
Once you have the DRD of the rest mass of an electron, it is sufficient to create an BH in 2D. The mass in 3D is not the same mass from itself in 2D, because the number of spatial dimensions involved in the mass is different. According to GR, this BH cannot form in 2D by a pure intrinsic mapping. An extrinsic mapping is needed again. In 3D, nothing else could be determined in the volume of the DRD. The BH gives the 2D image a higher-dimensional transition and occupies all spatial dimensions again in 3D. The trick is that a 2D image occupies all spatial dimensions in 3D through the singularity in a BH and thus has a rest mass.
However, the illustration is not a full wave. This corresponds to the given image. This is often used to explain gravity in 3D. There the picture is wrong. It explicitly shows a mixture of extrinsic and space curvature. Wrong in 3D, exactly right in 2D intrinsic.
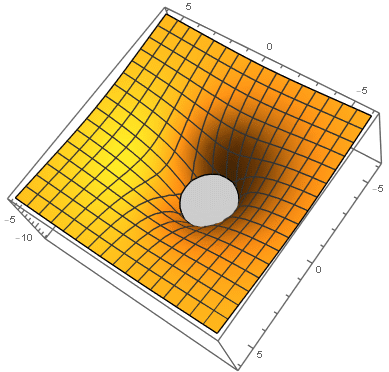
It is only half a wave from the point of view of a wave representation. Therefore, the spin is only ½ for this mapping. Since the mapping does not balance in 2D, the volume of space is “occupied”. No two equal mappings of this type can be placed in the same volume of space. The BH creates a constant gravitational field in 2D. Therefore, an electron has an electric field and is the source of the field. More detailed explanation at the Standard Model.
Quantisation
Quantisation generally has two characteristics. One is that energies actually only exist in certain levels and another is that the energy can be arbitrary, but the energy must always enter an interaction completely or not at all.
The simpler part is all or nothing in the interaction. The DRD expressions are always in 2D. There in different spacetimes. Spacetimes cannot “cut off” anything from each other. Neither 3D to 2D nor 2D to each other. This is especially not possible because time is not divided. An interactions cannot stop after “half the time”. Here 3D is the “collection pot” for all lower-dimensional expressions. It all goes in and is all distributed out again in the interaction.
For the quantisation, that the DRD expression itself can only take certain levels, the lower-dimensional expression is again responsible. Whether half or whole wave, the representation is always a wave. Otherwise, a 2D expression is not recognisable in the 3D volume. Now the wave must start and end at zero. The volume could only change if the amplitude were to change. But this is fixed by the interaction. Thus, only a half or a full wave can be introduced into this volume. It follows that the representations in 2D themselves form a potential well. In 3D alone there is no reason for quantisation. This only occurs via the dimensional transition.
In DP, no further fields are needed in addition to spacetime. The difference lies only in the respective geometric expressions in the different space dimensions. More on this in the chapter Standard Model.
Superposition, probability and collapse
Since there are infinitely many low-dimensional spacetimes, there can be infinitely many expressions for a single DRD. An infinite number of real expressions from the lower dimensional ones results in infinite energy. This is not observed. Here it is important to remember again that the DRD itself is in 3D with a concrete expression. In the lower dimensional, the DRD has infinite possibilities of a real expression. In the first approach, the DRD has no reason to choose an expression. As long as no concrete expression is selected, all possibilities exist simultaneously in the low-dimensional from the 3D view. The spacetimes are connected to space and not to spacetime. There is no temporal problem that all expressions are possible at any time. Thus the representation of the DRD in the low-dimensional is a superposition of all possible expressions for all possible states. Only with an interaction must an expression be connected with 3D for the matching geometry of the interaction. If at least two 2D expressions want to influence each other, this is only possible in 3D. Therefore, one can only obtain a special mapping from all possible mappings with a probability.
An interaction has a concrete geometry. For example, the photon is a transverse 2D wave of spacetime itself. If a DRD has no possible expression for this geometry, it cannot participate in the interaction, here e.g. a surface. The possibilities must commit to a concrete expression of this geometry. The interaction as DRD overlaps with another DRD and must react to it. The DRD takes the interaction and creates a new state. The determination happens in the collection pot 3D and is thus not a process in the lower dimensional. Therefore, for example, the Schrödinger equation cannot describe the “wave collapse”. The 3D determination must only be unique within the geometry required by the interaction. Therefore, in the case of a particle, only those properties are determined which are specified by the interaction.
If the determination of the property is bound to the 3D space via information, this property is part of the 3D DRD and cannot change again immediately. If a certain geometry (“property”) is determined, this geometry is observed again in a second measurement.
Entanglement
Entanglement is very easy to understand in DP. The crucial idea here is that entanglement is not an exchange of DRD in 3D spacetime.
An interaction is triggered by a DRD in 3D. The interaction itself is a DRD that exists in 3D spacetime. It is only at this level that entanglement cannot be understood. A DRD has a manifestation and thus property in the lower dimensional. Two different objects with the same wave function have this property in the same low-dimensional geometry. Thus, from the point of view of 3D and 2D, no distinction is possible in the wave description. These characteristics must be described with a single wave function. These objects can be brought to any distance in 3D in any time. This spacetime does not exist for the common low-dimensional geometry.
For entanglement, therefore, the following compelling conclusions can be drawn:
- Entanglement is low-dimensional, an interaction is connected to 3D spacetime. Therefore, no interaction in 3D is exchanged during entanglement.
- Since entanglement is unaware of 3D spacetime, any change to the low-dimensional geometry must happen instantaneously throughout the 3D universe. There is explicitly no delay possible.
- Since only the property is in the lower dimensional and information is bound to a 3D spacetime, no information can ever be transmitted faster than the SL via entanglement.
The problem of “spooky action at a distance” does not exist because there is no “distance” for the wave description of the entangled objects.
Indeterminacy
For an explanation of where the indeterminacy comes from, the classical example with momentum and location (\Delta p\space *\space \Delta x) is used. The momentum is a direct density in spacetime. If a density is to be measured, one necessarily needs a volume/distance. If the volume is made smaller in order to better determine the location, the density will always be more difficult to determine in relation to the rest. If, on the contrary, one wants to determine the density exactly, one would have to do this in relation to the entire remainder. In this case, the rest is spacetime itself.
For this measurement, the required amount of spacetime is opposite. Therefore, one does not get below a certain limit in the measurement. Since everything in DP is a mapping in spacetime, only a few combinations of measurements can be made exactly. The indeterminacy is already contained due to the definition of the DRD as space density.
Vacuum fluctuation
In the DP, a real vacuum with zero energy content cannot exist. At every point in spacetime, a “space density” and thus a non-zero DRD is given simply by the existence of the spacetime point. A point in space with no additional DRD is simply set as the zero level of the vacuum. There is always DRD/energy in the vacuum because there is always spacetime.
In QFT, every point in space has vacuum fluctuation due to its vacuum energy and indeterminacy. Here, there is a difference in the view between QFT and DP. Thus also a different statement on vacuum fluctuation. In QFT, the value zero may not be reached exactly due to the indeterminacy. The value may deviate positively or negatively from zero, but not exactly zero. In DP, zero is not attainable because there is a point in space. It is not possible to go from positive energy to zero. It is described in this chapter that the DRD needs a reason, in the form of an interaction (geometry) between DRDs, to get from the probability statement to a concrete expression of the geometry. In the DP, the spatial point remains in the superposition and thus does not generate a fluctuation. An “incentive/geometry” is needed. In the DP, fluctuation only occurs if there is already a DRD with at least one geometric expression. Gravity does not trigger fluctuation because it is in 3D and does not contain geometry for 2D.
Fluctuation only occurs when a DRD is present. The difference between DP and QFT cannot be determined by a direct measurement (Casimir effect), since a DRD is always present during a measurement. Indirectly, this is possibly possible through the vacuum energy of the entire spacetime. See the chapter on cosmology.
Hilbert space and QFT
Since the DRD has a geometric mapping, a vector space is a suitable mathematical basis for the description. If exactly one geometric expression is chosen, none of the other possibilities can have a share. Thus, the vector space is necessarily orthogonal and the scalar product must be defined.
If one wants to change from one state description to another state description, they have the same geometric basis. This geometric expression must not change, as it defines the DRD. Thus, this operator must necessarily be unitary.
There must be a sub-vector space, since the geometry is not completely determined for every interaction. The more the geometry is to be determined, the more information is needed in the wave equations. For every possible single geometry. One goes from the Schrödinger equation to the Pauli equation and then to the Dirac equation.
The Schrödinger equation must have a complex representation. The wave described has an expression in 3D and in the lower dimension. Therefore, one needs two different “levels” of representation in one equation. This is what the complex numbers bring.
In QFT, the operators create and annihilate the objects. This corresponds to the real representation better than the operators in quantum mechanics. The reason is that the DRD is in 3D and the interaction and the object pay into this “collection pot” and a new object is formed with the changed properties. The 3D DRD is the “mediator” between the lower dimensional mappings.
The path integral method in QFT is the correct mathematical calculation for any particle. The low-dimensional expression is connected via space and not spacetime. A particle thus has “infinite” time to actually do anything. In addition, this DRD has all possible low-dimensional spacetimes for a movement in its own spacetime. As crazy as the idea may sound. The particle can, without a restriction in 3D (e.g. a wall), test out the entire 3D spacetime in “zero time”. Only the sum of all possibilities must then again adhere to the limitations from 3D.
Quantum mechanics and the OFT are indeed the physically correct descriptions for the real representation. It is not just a mathematical auxiliary construct that delivers the appropriate result.